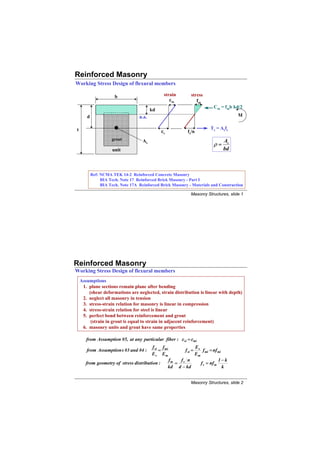
Lecture 6 7 Rm Shear Walls

Reinforced Masonry
Working Stress Design of flexural members

b strain stress
εm fm
Cm = fmb kd/2
kd
d n.a. M

Ts = Asfs
t εs fs/n
grout As As
ρ=
unit bd

Ref: NCMA TEK 14-2 Reinforced Concrete Masonry
BIA Tech. Note 17 Reinforced Brick Masonry - Part I
BIA Tech. Note 17A Reinforced Brick Masonry - Materials and Construction

Masonry Structures, slide 1

Reinforced Masonry
Assumptions
1. plane sections remain plane after bending
(shear deformations are neglected, strain distribution is linear with depth)
2. neglect all masonry in tension
3. stress-strain relation for masonry is linear in compression
4. stress-strain relation for steel is linear
5. perfect bond between reinforcement and grout
(strain in grout is equal to strain in adjacent reinforcement)
6. masonry units and grout have same properties

from Assumption #5, at any particular fiber : ε si = ε mi
f si f mi Es
from Assumption s #3 and #4 : = f si = f mi = nf mi
E s Em Em
fm f n 1− k
from geometry of stress distribution : = s f s = nf m
kd d − kd k


Reinforced Masonry
from equilibrium, C = T :
f m bkd 1−k
= As f s = ρ bd f s = ρ bd n f m
2 k
1− k
k / 2= ρ n k 2 + 2 ρnk − 2 ρn = 0
k
from equilibrium: ∑ M about C m = 0
M s = As f s jd = ρbd 2 jf s where jd=d-kd/3 or j=1-k/3

If fs=Fs then moment capacity will be limited by reinforcement.

Allowable reinforcement tensile stress per MSJC Sec.2.3.2:
Fs=20 ksi for Grades 40 or 50; Fs=24 ksi for Grade 60
Fs=30 ksi for wire joint reinforcement
Allowable reinforcement tensile stress per UBC Sec.2107.2.11 :
Fs= 0.5fy < 24 ksi for deformed bars; Fs= 0.5fy < 30 ksi for wire reinforcement
Fs= 0.4fy < 20 ksi for ties, anchors, and smooth bars


Reinforced Masonry

from equilibrium: ∑ M about T s =0

M m = 0.5 f m bkdjd = 0.5 f m jkbd 2

If fm= Fb then moment capacity will be limited by masonry.
UBC 2107.2.6 & MSJC Sec.2.3.3.2:
Fb=0.33f’m


Reinforced Masonry
WSD: Balanced Condition
fm = F b

Definition: The balanced condition occurs kbd
C = fmb kd/2
when the extreme fiber stress in the masonry is
equal to the allowable compressive stress, Fb, d
and the tensile stress in the reinforcement is
equal to the allowable tensile stress, Fs.
T = As fs
fs/n = Fs /n

• For any section and materials, only one unique amount of balanced
reinforcement exists.
• Although balanced condition is purely hypothetical case, it is useful because it
alerts the engineer to whether the reinforcement or the masonry stress will
govern the design. Balanced stresses are not a design objective.


Reinforced Masonry
WSD: Balanced Condition
from geometry: from equilibrium: C=T
Fs
fm = Fb Fb +
Fb n d
= Fb b kb = ρb b d Fs
kb d d 2
kbd
Fk F n
d Fb ρb = b b = b
kb = 2 Fs 2 Fs ( n + Fs )
Fs
Fb + Fb
n
fs/n = Fs /n
n ⎡ n ⎤⎡ 1 ⎤
kb = ρb = ⎢ ⎥⎢ ⎥
n + Fs /Fb ⎣ n + Fs / Fb ⎦ ⎣ 2Fs / Fb ⎦


Example: Balanced Condition
Determine the ratio of reinforcement that will result in a balanced condition per UBC.
Given: f’m = 2000 psi and Grade 60 reinforcement

Fb = 0.33 f'm = 667 psi Fs = 24 ksi for Grade 60 reinforcement
E m = 750 f'm = 1500 ksi E s = 29,000 ksi

E s 29,000
n = = = 19.3
Em 1500

⎡ 19.3 ⎤⎡ 1 ⎤
ρb = ⎢ = 0.48%
⎣ 19.3 + 24/0.667 ⎥ ⎢ 2 x24/0.667 ⎥
⎦⎣ ⎦


Design Strategy for RM Flexural Design
Procedure for sizing section and reinforcement for given moment.

Calculate ρb knowing f’m and fy
determine Fb from f’m
determine Fs from fy
determine Emfrom f’m
determine n = Es/Em

Size section for some ρ < ρb Note: Section must also be
determine k and j sized for shear.
bd2 = M/ρjFs
select b and d using common units

Size reinforcement Check design
As = M/Fsjd Ms = AsFsjd > M
select number and size of rebars fb = M/0.5jkbd2 < Fb


Example: Reinforced Masonry
Design a beam section for a moment equal to 370 kip-in. Prisms have been
tested and f’m is specified at 2000 psi. Use Grade 60 reinforcement and 8”
CMU’s.
1. From previous example, ρb = 0.48%
2. Estimate ρ to be slightly lower than ρ so steel will govern.
b
A good estimate is ρ = 0.4%
3. Solve for k :
k 2 + 2 ρnk − 2 ρn = 0
2 ρn = 2( 0.004 )( 19.3 )= 0.154
k 2 + 0.154 k − 0.154 = 0
k = 0.323 j = 1 − k / 3 = 0.892
4. Solve for bd 2 :
bd 2 = M / ρ j F s
bd 2 = ( 370 kip − in ) /( 0.004 )( 0.892 )24 ksi ) = 4321 in 3


Example: Reinforced Masonry
5. Select dimensions of beam using 8” CMU’s:
b = 7.63” 4 - 8” CMU’s
dreq’d = [4321 / 7.63]0.5 = 23.8” d=27.8”
use four units and center bars in bottom
unit, d = 27.8”
6. Estimate amount of reinforcem ent :
As req' d = M / Fs j d 7.63”

As req' d = (370 kip - in) / (24 ksi) (0.892) (27.8) = 0.62 in 2
use 2 #5' s (0.62 in 2 )

7 . Check design:
ρ = Αs / bd = 0.62 in 2 / (7.63quot; ) ( 27.8quot; ) = 0.00292
k 2 + 2 ρnk − 2 ρn = 0 k = 0.284 j = 1 − 0.284 / 3 = 0.905
Μ s = Αs Fs j d = ( 0.62 in 2 )( 24 ksi )( 0.905 )( 27.8quot; ) = 374 kip − in. > 370 kip − in. OK
( 370 kip − in x 1000 )
f m = Μ / 0. 5 j k d 2 = = 488 psi < 667 psi ok
( 0.5 )( 0.905 )( 0.284 )(7.63quot; )( 27.8quot; ) 2


Flexural Capacity of Partially Grouted Masonry
Case A: neutral axis in flange * per MSJC Sec. 2.3.3.3
flange
b = 6t or 72” or s* b
tf kd
neutral
d
t axis
As
As per width b

If neutral axis is in flange, cracked section is the same as a solid rectangular
section with width “b.” Therefore, depth to neutral axis from extreme
compression fiber may be calculated using:
As
k 2 + 2 ρnk − 2 ρn = 0 ρ=
bd

If kd < tf assumption is valid, determine moment capacity as for rectangular section.
If kd > tf assumption is not valid, need to consider web portion.


Shear Design of Reinforced Masonry
s
Cm
Vm
d
Vs Vext.
Viy
Asfs
Vd
R Basic shear mechanisms:
before cracking: Vext = Vint = Vm + Vd + Viy + Vs
Once diagonal crack forms:
• Vm reduces • flexural stresses increase
• dowel action invoked • fsa is related to Mb

Presence of shear reinforcement will:
• restrict crack growth • resist tensile stress
• help dowel action



after cracking: Vext = Vint = Vs = nAvfs where n is the number of transverse
bars across the diagonal crack.
Assuming a 45 degree slope, n=d/s
Vs = (d/s)Avfs

Av Vs Vs UBC Sec. 2107.2.17 (Eq. 7.38)
= = MSJC Sec. 2.3.5.3 (Eq. 2-26)
s df s dFs


Shear Design of Reinforced Masomry
Flexural shear stress
dx
C
M C + dC M + dM fvbdx
na
jd

T T + dT T T + dT

fvb dx = dT = dM/jd
fv = (dM/dx)/bjd

V
fv = UBC Sec. 2107.2.17 (Eq. 7-38)
bjd
V
fv =
bd MSJC Sec. 2.3.5.2.1 (Eq. 2-19)


Allowable shear stresses for flexural members per UBC and MSJC

UBC Sec. 2107.2.8.A and MSJC Sec. 2.3.5.2.2(a):
members with no shear reinforcement
Fv = 1.0 f'm < 50 psi UBC Eq. 7-17; MSJC Eq. 2-20

UBC Sec. 2107.2.8.B and MSJC Sec. 2.3.5.2.3(a):
members with shear reinforcement designed to take the entire shear

Fv = 3.0 f'm < 150 psi UBC Eq. 7-18; MSJC Eq. 2-23


Allowable shear stresses for shear walls per UBC and MSJC

UBC Sec. 2107.2.9.i and MSJC Sec. 2.3.5.2.2(b):
walls with in-plane flexural reinforcement and no shear reinforcement
M 1 M M
for <1 F v = ( 4 − ) f 'm <( 80 − 45 ) psi UBC Eq. 7-19; MSJC Eq. 2-21
Vd 3 Vd Vd
M
for ≥1 F v = 1.0 f 'm < 35 psi UBC Eq. 7-20; MSJC Eq. 2-22
Vd

UBC Sec. 2107.2.9.ii and MSJC Sec. 2.3.5.2.3(b):
walls with in-plane flexural reinforcement and shear reinforcement designed
to take 100% of shear
M 1 M M
for <1 Fv = ( 4 − ) f'm < ( 120 − 45 )psi UBC Eq. 7-21; MSJC Eq. 2-24
Vd 2 Vd Vd
M
for ≥1 F v = 1.5 f 'm < 75 psi h UBC Eq. 7-22; MSJC Eq. 2-25
Vd


Moment-to-Shear Ratios

For a single-story For piers between openings
cantilevered shear walls
M
d
V V

d
h h

M M

M Vh h M Vh / 2 h
= = = =
Vd Vd d Vd Vd 2d


Additional MSJC Requirements

MSJC Sec. 2.3.5.3.1
smax = d/2 or 48”
MSJC Sec. 2.3.3.4.2
minimum reinforcement perpendicular to shear reinforcement = Av/3
smax = 8 ft

MSJC Sec. 2.3.5.5
design for shear force at distance “d/2” out from support

Vdesign Vdesign

d/2


Shear Design Strategy for Reinforced Sections

Start

Determine Flexural Tension Stress Determine Fv Assuming Shear
ft= -P/A+Mc/I Reinforcement to take 100% of Shear

consider as no no
is ft>Ft? Resize
unreinforced is fv<Fv?
Section
yes
yes
Determine Maximum Design Shear
Provide Reinforcement to Take
100% of Shear
Determine Shear Stress Av V
V V = s
fv = or s dFs
bjd bd no
yes
Determine Fv Assuming No Shear is fv<Fv? End
Reinforcement


Example: Design of RM Shear Wall
Determine the maximum lateral force, Hwind per UBC and MSJC

6’-8” 8” CMU wall
120 psi Type S - PCL mortar
solidly grouted f’m=3000 psi

#4 @ 32”

2 - #8’s each end of wall
8’-0”

Case A: neglect all reinforcement
Case B: consider vertical reinf., neglect horizontal reinf.
Case C: consider vertical and horizontal reinf.
6’-4” Case D: design horizontal reinforcement for max. shear


Case A: neglect all reinforcement
per UBC: 7.63 × 80 2
Sg = = 8139 in3
flexure 6
M 96 × H
- fa + = Ft − 120 + = 40 x 1.33 H = 14 ,684 lbs. = 14.7 kips
S 8139
shear
Fv = [ 34 psi + 0.2 fa dead ] x 1.33 = [ 34 + 0.2 ( 120 )] x 1.33 = 77.1 psi
77.1 psi
Vmax = Ae Fv = ( 7.63 x 80.0 )( ) = 47.1 kips
1000
per MSJC:
flexure 96 × H
- fa + M / S = Ft − 120 + =0 H = 10 ,174 lbs . = 10.2 kips
shear 8139
Fv = 60 + 0.45 ( 120 ) = 114 psi > 1.5 f'm = 82.2 psi
Fv = 1.33 × 82.2 psi = 109 psi
2 2
Vmax = Fv bt = ( 109 psi )( 7.63 x 80 ) = 44.3 kips
3 3

Case B: consider only vertical reinforcement
Flexure by UBC or MSJC: neglecting fa
Ms = AsFsjd = 2 x 0.79 in2 (1.33 x 24 ksi) (0.9 x 72.0”) = 3268 k-in Hwind = 34.0 kips
lumping 2 - #8’s ave. d for 2 bars

Shear per UBC Sec.2107.2.9 or MSJC Sec.2.3.5.2
8.0'
M/Vd = = 1.33 > 1
6.0'
M
for > 1 Fv = 1.0 f 'm < 35 psi Fv = 1.33 x 35 psi = 46.6 psi
Vd

for UBC Vmax = bjdFv = ( 7.63quot; )( 0.9 )( 72quot; )( 46.6 psi ) / 1000 = 23.0 kips governs

for MSJC Vmax = bdFv = ( 7.63quot; )( 72quot; )( 46.6 psi ) / 1000 = 25.6 kips governs


Case C: consider all reinforcement
Flexure by UBC or MSJC: same as case B
Shear per UBC Sec. 2107.2.17 or MSJC Sec.2.3.5.3
Vmax= Vs=(Av/s)Fsd = (0.20 in2/32”)(24 ksi x 1.33)(72”) = 14.4 kips governs

Overall shear per UBC Sec. 2107.2.9.C or MSJC Sec. 2.3.5.2.3 (b)
M
for > 1 F = 1.5 f'm ≤75 psi F = 1.5 3000 = 82.2psi>75 psi
v v
Vd
Fv = 1.33x 75 psi= 100 psi

V (14.4 kips x 1000)
UBC f v = = = 30.7 psi < 100 psi okay
bjd (7.63)( 0 .9 )(72)

V (14.4 kips x 1000)
MSJC f v = = = 27.7 psi < 100 psi okay
bd (7.63)(72)


Case D: design horizontal reinforcement for maximum shear strength
Vmax = Fvbjd = ( 100 psi)( .63)(0.9 )(72)/ 1000 = 49.4 kips > 34 kips oka govern
7 y s
Av /S = Vmax/Fsd = 49.4 kips/( .33 x 24 ksi)( ) = 0.0215in2 per in.
1 72
u sing #4 rebars(Av = 0.20 in2 ) s = 0.20 / 0.0215 = 9.3quot; use # @ 8 in. horiz
4 ontal
Summary: Hmax, kips

Case Consideration UBC MSJC
A No steel
No steel 14.7*
14.7* 10.2*
10.2*
vertical steel 24.3 27.0
B vertical steel 23.0 25.6
no horizontal steel
no horizontal steel
vertical steel and 15.2 15.2
C vertical steel and 14.4 14.4
#4 @ 32” horizontal steel
#4 @ 32” horizontal steel

D #4#4 @ 8” horizontal
@ 8” horizontal 34.0*
34.0* 34.0*

*flexure governs


Flexural Bond Stress
M = Tjd
M + dM = (T + dT)jd
dM = dT jd
dx dT = dM/jd

C + dC U = bond force per unit length for group of bars
C
U dx = dT = dM/jd
U = (dM/dx)/jd = V/jd
M U
dx jd u = flexural bond stress =
M + dM ∑o
where ∑ o = sum of perimeters of all bars in group
T T + dT
V
u= UBC Sec. 2107.2.16 Eq. 7-36
dx Σ ο jd
U
T + dT allowable bond stress per UBC Sec.2107.2.2.4:
T 60 psi for plain bars
200 psi for deformed bars
dx 100 psi for deformed bars w/o inspection


Development Length

uπ d b

db As fs

ld

As fs = uπdb ld
πd b
2
fs = uπdb ld
4
f s db
ld = = 0.002 db fs for u = 125 psi UBC Sec. 2107.2.2.3 Eq.7 - 9
4u
ld = 0.0015 db Fs for u = 167 psi MSJC Sec . 2.1.8.2 Eq . 2 − 8


Embedment of Flexural Reinforcement
UBC Sec. 2106.3.4 and MSJC Sec.2.1. 8.3
Rule #1: extend bars a distance of “d” or “12db” past the theoretical cutoff point
Rule #2: extend bars a distance of “ld” past the point of maximum stress
Example for shear wall:

bars “a”
Moment Diagram
(#2)
> ld
(#1)
d or 12db

theoretical cutoff point
capacity with bars “a”
bars “b”

(#2)
> ld
moment capacity
with bars “a” and “b”

Combined Bending and Axial Loads
Code Requirements
UBC Sec. 2107.1.6.3 fa fb
use unity formula to check compressive stress: + < 1.0
Fa Fb
UBC Sec. 2107.1.6.1 UBC Sec. 2107.2.15
P M Note: unity formula is conservative -
better approach is to use P-M
interaction diagram.

M
As fs fb = ( Eq .7 − 31 )
2 jkbd 2
fa = P/Ae kd
jd
UBC Sec. 2.14.2
if h’/t >30 then analysis should consider effects of deflections on moments

MSJC Sec. 2.3.3.2.2 fa + fb < 1/3 f’m provided that fa < Fa
In lieu of approximate method, use an axial-force moment interaction diagram.


Axial Force-Moment Interaction Diagrams
General Assumptions
1. plane sections remain plane after bending
• shear deformations neglected
• strain distribution linear with depth
2. neglect all masonry in tension
3. neglect steel in compression unless tied Strain Stress
4. stress-strain relation for masonry is linear in compression εm fm
5. stress-strain relation for steel is linear
6. perfect bond between reinforcement and grout Cs
• strain in grout is equal to strain in adjacent
reinforcement
7. grout properties same as masonry unit properties

P

M
εs
Ts=Asfs


Axial Force-Moment Interaction Diagram
Out-of-Plane Bending of Reinforced Wall
Pa
Mb

d = t/2
Range “a”:
large P, small M, e=M/P < t/6
unit width = b

Pa = 0.5(fm1 + fm2)A

Ma= 0.5(fm1 - fm2)S where S = bt2/6

fm2
fm1
em
Cm


Pb
Mb
d = t/2 Range “b”
medium P, medium M, e > t/6, As in compression
unit width = b

0.5 < α < 1.0 for section with reinforcement at center
t αt
em = −
2 3
fm 1
fm1 Pb = C m = αtb
em 2
Cm
M b = C m em
αt


Pc
Mc
Range “c” small P, large M, e > t/6, As in tension
d = t/2

α < 0.5 for section with reinforcement at center
unit width = b

t αt
em = −
2 3
fm 1
Pc = C m − Ts Cm = αtb Ts = As fs
2

f s ⎡ d − αt ⎤ ⎡ 0. 5 − α ⎤ t
= fm 1 = ⎢ f for d =
fm1 n ⎢ αt ⎥
⎣ ⎦ ⎣ α ⎥ m1
⎦ 2
em
Ts
Cm t
M c = C m em + Ts ( d − )
αt 2


Range “a” Range “b” Range “c”
e=0; M=0
fm1= fm2=Fa fm1= Fb= f’m/3
P=Fa A
Reduce fm2 from Reduce α from Reduce α from
2Fa-Fb by 1.0 by 0.5 by
Start increment increment increment

compression controlling
Determine P & M Determine P and Determine P and

tension controlling
per Range “a” M per Range “b” M per Range “c”

no yes no is As in yes yes
fm2 = 0? tension? M = 0?

no
no yes
fs = Fs fs < Fs? fs < Fs
fm1 < Fb fm1 = Fb

Stop



fm1 = Fb
Fb
fm1 = Fa fm2 = 2Fa - Fb
Fa Fb Range “a”
lim
it b
yu
Axial Force

Fb Range “b”
nit

1
y
for

tension compression

e
mu

fs/n
controls controls

Fs/n
la

Fb Fb

balanced point Range “c”

Fs Fs/n Moment
fs =
fm


Example: Interaction Diagram
Determine an axial force-moment interaction diagram for a fully grouted 8” block
wall reinforced with #4 @ 16”. Prism compressive strength has been determined by
test to be equal to 2500 psi. Reinforcement is Grade 60. Height of wall is 11.5 feet.

Fs = 24 ksi for Grade 60
Fa = 0.25 f´ m = 625 psi without reduction factor

Fb = 0.33f´ m = 833 psi
E m = 750 f´ m = 1875 ksi per UBC E s = 29,000 ksi
n = E s /E m = 15.5
per foot of wall : Ag = 7.63quot; x 12quot; = 91.6 in 2 ; S g = 12quot; x 7.63 2 / 6 = 116 in 3
As / ft = 0.20 x 12 / 16 = 0.150 in 2
ρ = 0.20 in 2 /( 16 x 3.81quot; ) = 0.0033 ρn = 0.0509 k = 0.272 j = 0.909


Fs ⎛ α ⎞
*masonry stress inferred from Fs and α: f m 1 = ⎜ ⎟
n ⎝ 0.5 − α ⎠

Range Case fm1 fm2 α Cm em Ts P=Cm- Ts M=Cm em
(psi) (psi) (kips) (in.) (kips) (kips) (kip-in)
1 625 625 - 57.2 0 - 57.2 0
Compression Controls

a 2 833 417 - 57.2 - - 57.2 24.1
3 833 0 - 38.1 1.27 - 38.1 48.4
4 833 - 0.75 28.6 1.91 - 28.6 54.5
b 5 833 - 0.50 19.1 2.54 - 19.1 48.5
6 833 - 0.33 12.6 2.97 0.9 11.7 37.4
7 833 - 0.25 9.5 3.18 2.0 7.5 30.2
8 833 - 0.167 6.4 3.39 3.9 2.5 21.5
9 for P = 0: Mm= 0.5Fbjkbd2 = 0.5(833 psi)(0.909)(0.272)(12)(3.81)2 = 17.9
c
Controls

10 833 bal. - 0.175 6.7 3.37 3.6 3.1 22.5
Tension

11 664* - 0.150 4.6 3.43 3.6 1.0 15.7
12 check for P = 0: Ms = AsFsjd = (0.15 in2)(24 ksi)(0.909)(3.81”) = 12.5


50 1 2 6 833 0.9k
1 625
0.33t

40 2.0 k
2 833 7 833
Axial Force 3 417 0.25t
kips
30 4 0
3 833 8 833
3.9 k > AsFs
20
5 0.167t
4 833 10 833
6 .75t 3.6 k = AsFs
10
10 0.175t
11 7
12 5 833 11 664
3.6 k = AsFs
10 20 30 40 50 0.15t
8 0.50t
9
Moment, kip-in


Flexural Capacity with Axial Compression
Short Cut Method
Out-of-Plane Bending, Reinforcement at Center
fm
d kd Cm

d
jd
M
Ts P
fs/n

f s /n f k f E
stress compatibility: = m ; fm = ( s ) where n = s [1]
d - kd kd 1-k n Em
C m = 0.5 f m bkd [2]

Ts = As f s = ρbdf s [3]


Flexural Capacity with Axial Compression
Short Cut Method
P = C m - Ts [4]
equilibrium:
P = 0.5 f m bkd - ρbdf s [5]
f ⎛ k ⎞
P = 0.5 ( s )⎜ ⎟ bkd - ρbdf s [6]
n ⎝1-k ⎠
P k2 ⎛ 1 ⎞
= 0 .5 ⎜ ⎟− ρ [7]
bdf s 1− k ⎝ n⎠
P
if tension controls , f s = Fs set α = [8]
bdFs
2
k ⎛ 1⎞
a = 0.5 ⎜ ⎟− ρ [9]
(1 − k) ⎝ n ⎠
1 k2
ρ +α = [10]
2n 1 − k
k 2 + 2 n( ρ + α )k − 2 n( ρ + α ) = 0 [11]

M = C m jd = 0.5 f m bkjd 2 where j = 1 − k / 3 [12]


Strength Design of Reinforced Masonry
Ultimate Flexural Strength
strains stresses
As ε mu
Cm
c
d n.a.
t
d Mn

b
εs > εy Ts = Asfy

k3f’m k3f’m
fm f’m
k2c k2c
c klc
Cm = c
Cm = k1k3f’mbc
εm
εmu
Note: rectangular stress block can represent
compressive stress distribution if k2/k1 = 0.5

Measuring k1k3 and k2
Po P1 Po in displacement control
P1 in force control

summing moments about centroid:
a
increase P1 P1a = (Po + P1)g
so that ∆ = 0 = (Po + P1)(c/2 - k2c)
∆
P1 a
k 2 = 0.5 -
c Po + P1 c
strain
g total compressive force:
stress Po + P1 = k3f’m k1cb
P0+P1 Po + P1
k2c k1 k 3 =
f 'm bc
k3f’m
k1c


Measured k1k3 and k2 values

Sample experimentally determined constants k1k3, and k2
1

0.8
K1K3
K1K3 & K2

0.6

0.4
K2
0.2

0
0 0.001 0.002 0.003 0.004 0.005 0.006
Extreme Fiber Strain (in/in)


Ultimate Flexural Strength
equilibrium
C m + Ts = 0
k1 k 3 f 'm bc = As f y = ρbd f y fs
ρdf y fy
c =
k1 k 3 f 'm
summing moments about Cm
εs
M n = As f y ( d − k 2 c )
ρ df y
M n = As f y ( d − k 2 )
k 1 k 3 f 'm
ρf y
M n = As f y d ( 1 − k 2 )
k 1 k 3 f 'm
k2 fy
if = 0.5 and k 3 = 0.85 then : M n = As f y d ( 1 − 0.59 ρ )
k1 f 'm


Balanced condition with single layer of reinforcement

strains stresses strain compatibility
ε mu c ε mu
ε mu = or c = d
ε mu + ε y d ε mu + ε y
k1c Cm
c equilibrium
n.a. C m + Ts = 0
d Mn ε mu
k1 k 3 f 'm b d = ρ b bdf y
ε mu + ε y

k1 k 3 f 'm ε mu
ρb =
εs = ε y
Ts= Asfy fy ε mu + ε y

fy
if k 3 = 0.85 ε mu = 0.003 ε y = E s = 29 ,000 ,000 psi :
Es
k1 ( 0.85 ) f 'm 0.003 0.85 k1 f 'm 87 ,000
ρb = =
fy 0.003 + f y / E s fy 87 ,000 + f y


Balanced condition with single layer of reinforcement

0.85 k1 f 'm 87 ,000
ρb = ρ tb = As / bt for one layer of steel t = 2d k 1 = 0.85
fy 87 ,000 + f y

Grade 40 Grade 60
f’m ρb ρ tb ρb ρ tb

1000 0.0124 0.0062 0.0071 0.0036
2000 0.0247 0.0124 0.0143 0.0072
3000 0.0371 0.0186 0.0214 0.0107
4000 0.0495 0.0247 0.0285 0.0142
5000 0.0619 0.0309 0.0356 0.0178
6000 0.0742 0.0371 0.0428 0.0214


Balanced condition with multiple layers of reinforcement
strains stresses
b
ε mu 0.85f’m
d1 ε s1 Cs1
d2
0.85c

ε s2 Cm=0.85f’mb(0.85c)
Cs2
c
d3
d4

ε s3 Ts3
Ts4 = Asbal fy
ε s4 = ε y
Asbal

strain compatibility equilibrium
d ε mu f si = E sε si < f y
ε si < ε mu − ( i )( ε mu + ε sy ) c= d
d4 ε mu + ε y C si or Tsi = Asbal i f si
60 ksi C m + ∑( C si + Tsi ) = 0
εy= = 0 .00207 (Grade 60)
29,000 ksi − 0.428 f 'm bd + Asbal ∑ f si = 0
if ε mu = 0.003 , then c = 0 .592 d
solve for Asbal

Example: Flexural Strength of In-Plane Wall
Determine the maximum bar size that can be placed as shown.
Maximum steel is equal to one-half of that resulting in balanced conditions.
f’m= 1500 psi Grade 60 reinforcement special inspection

7.63”
0.003 0.85f’m
ε s1
4.0” Cs1
20.0” Cm = 0.85f’mb(0.85c)
εs 2
44.0”
c
60.0” Cs2
5’-4”

Pn = 0
n.a.
εs 3 Ts3
Asbal ?

Ts4 = Asbal fy
ε s = ε y = 0 .00207

Determine the maximum bar size.
c = 0.003/0.00507 (60.0”) = 35.5”
Cm = 0.85f’mb(0.85c) = -0.85(1500)(7.63”)(0.85 x 35.5) = -294 k

layer di εsi fsi
⎛ c − d1 ⎞
εi =⎜ ⎟ ( −0.003 )
⎝ c ⎠ 1 4.0” -0.00261 (C) -60.0
2 20.0” -0.00131 (C) -38.0
f si = E sε si ≤ f y 3 44.0” 0.00072 (T) 20.8
4 60.0” 0.00207 (T) 60.0
without compression steel (neglect Cs1 andCs2 forces)
Cm + Σ(Csi + Tsi) = -294 + Asbal (20.8+ 60.0) = 0 Asbal = 3.64 in2 Asmax = 1.82 in2
max. bar size is #ll (1.56 in2)*
*bars larger than #9 are not recommended because of anchorage and detailing problems

with compression steel (include Cs1 and Cs2 forces)
Cm + Σ(Csi + Tsi) = -294 + Asbal (-60.0 - 38.0 + 20.8 + 60.0) = 0 Asbal = -17.1 in2
note: negative Asbal means that ΣC > ΣT , in such case no limit on tensile reinforcement


Determine flexural strength of wall.
f’m= 1500 psi Grade 60 reinforcement special inspection

0.003 0.85f’m
ε s1 Cs1
4.0”
20.0”
c Cm = 0.85f’mb(0.85c)

44.0” n.a.
Ts2
60.0” εs 2
5’-4”

εs 3
Ts3

εs > ε y
Ts4 = As fy
#8 (typ) = 0.79 in2 x 60 ksi = 47.4 k
7.63”


Determine flexural strength of wall.
⎛ c − di ⎞
εi =⎜ ⎟( −0.003) compressive strains = (-) fsi = Esεsi < f y Cm =8.27c
⎝ c ⎠
d1 = 4.0” d2 = 20.0” d3 = 44.0”
c ε1 f1 Csl ε2 f2 Ts2 ε3 f3 Ts3 Cm ∑(C + T )

0.00360 60.0
20.0 -0.00240 -60 -47.4 0 0 0 -165 -117.6
47.4
15.0 -0.00220 -60 -47.4 0.00100 29.0 22.9 0.00580 60.0 47.4 -124 -54

11.0 -0.00191 -55 -43.7 0.00245 60.0 47.4 0.00900 60.0 47.4 -91 +7.5

11.5 -0.00196 -56 -44.8 0.00222 60.0 47.4 0.00848 60.0 47.4 -95 +2.3

0.85 c close to zero, take c = 11.5”
M n = ∑ { Asi f si ( d i − )}
2
= ( −44.8 )( 4.00 − 4.89 ) + ( 47.4 )( 20.0 − 4.89 ) + ( 47.4 )( 44.0 − 4.89 )
+ ( 47.4 )( 60.0 − 4.89 ) = 5 ,222 kip − in


Approximate flexural strength of wall.

neglecting C sl and Ts 2 , and lumping As 3 and As 4
( 60 + 44 ) 2 × 0.79
d= = 52.0quot; ρ= = 0.00398
2 7.63 × 52

fy
5’-4”

M n = As f y d ( 1 − 0.59 ρ )
f 'm
60
= 2( 0.79 in 2 )( 60 )( 52.0 )( 1 − 0.59 x 0.00398 x )
1.50
= 4 ,467 kip − in 86% of answer
#8 (typ)

7.63”


Slender Wall Design
Limitations of Method:
UBC Sec. 2108.2.4
(a) for out-of-plane bending of solid, reinforced walls lightly stressed under gravity loads
(b) limited to:
Pw + Pf
≤ 0.04 f'm ( 8 − 19 ) Sec. 8.2.4.4
210 where f'm <6000 psi
Ag
Pw + Pf h'
Note : when 0.04f'm < < 0.20f'm , method still can be used providing that < 30
Ag t
(c) ρg= As/bt < 0.5 ρbal
(d) special inspection must be provided during construction
(e) t > 6”
Sec. 2108.1.3: Load factors
U = 1.4 D + 1.7 L U = 0.9 D ± 1.4 E
U = 1.4( D + L + E ) U = 0.9 D ± 1.3W
U = 0.75( 1.4 D + 1.7 L + 1.7W )

Ref: NCMA TEK 14-11A Strength Design of Tall Concrete Masonry Walls


Slender Wall Design
Required Flexural Strength: UBC Sec. 2108.2.4.4
e eccentric transverse
P∆
load load
Puf
Pufe

t
h/2
Puw
wu
h
wuh2/8 (Puw + Puf)∆ u
Pufe/2
h/2

Pu = Puf + Puw
wu h2 Puf e
Mu = + + ( Puw + Puf )∆u ( 8 − 20 )
8 2


Slender Wall Design
Design Considerations

Design strength: Sec. 2108.2.4.4
Mu < φ Mn (8 - 22)

Strength reduction factor:
flexure φ = 0.8 Sec. 2108.1.4.2.1

Assumptions for ultimate flexural strength (Sec. 2108.2.1.2)
1. equilibrium
2. strain compatibility
3. εmu = 0.003
4. fs = Esεs < fy
5. neglect masonry strength in tension
6. rectangular stress block, k1 = 0.85, k3 = 0.85


Slender Wall Design
Equivalent area of reinforcement, Ae
for single wythe construction reinforced at center:
d
As a = 0.85c
b
0.85f’m
Ts = Asfy
d
c Pu
Cm =0.85f’mb(0.85c)

Ts = Asfy Pu = Cm - Asfy
Cm
Cm = Pu + Asfy= Asefy
( Pu + As f y )
Pu Ase = Eq. (8-24)
fy
flexural strength
a ( Pu + As f y )
M n = Ase f y ( d − ) Eq. (8 - 23) where a = Eq. (8 - 25)
2 0.85 f 'm b


Slender Wall Design
Lateral Deflections 5 wh 4 5 wh 2 h 2 5 Mh 2
∆= = =
M 384 EI 48 8 EI 48 EI

5 M s h2
for Ms < Mcr ∆s = (8-28)
My 48 E m I g
∆y 5 M cr h 2 5 ( M s − M cr )h 2
Ms for Ms > Mcr ∆s = + (8-29)
48 E m I g 48 E m I cr
∆s
Mcr b( kd )3
where I cr = nAse ( d − kd )2 +
∆cr 3
Mcr = fr S
∆
(note “kd” may be replaced by “c” for simplicity)

Modulus of Rupture, fr Eqs. 8-31, 32, 33
fully grouted partially grouted

hollow unit 4.0 f'm < 235 psi 2.5 f 'm < 125 psi

2-wythe brick 2.5 f 'm < 125 psi not allowed


Slender Wall Design
Design Considerations

Serviceability Criteria
∆s ≤ 0.007 h ( 8 − 27 )

Strength Criteria

w h 2 Puf e
Mu = u + + ( Puw + Puf )∆u
8 2

5 M cr h2 5 ( M u − M cr )h2
∆u = +


Example: Slender Wall Design
Determine the maximum wind load, w, per UBC and MSJC
3.5”
500 lbs/ft dead
200 lbs/ft live
3’-0”

P
w 8” CMU, partially grouted
f’m = 2000 psi, Grade 60
7 .63quot;
e = 3 .50quot; + = 7 .31quot;
2
12quot; in 2
As = 0 .20 in 2( ) = 0 .075
32quot; ft
20’-0”

#4 @ 32”

As 0 .075 1 1
ρ = = = 0 .000164 < ρbal = ( 0.0143)=0.0072 ok
bd 12× 3.81 2 2


Flexural Strength per UBC
U = 0.75 (1.4D + 1.7L + 1.7W)
w h 2 Puf e a
Mu = u + + ( Puw + Puf )∆u < φ M n = φ Ase f y ( d − )
8 2 2
Pu = Puf + Puw Puf = 0.75 ( 1.4 x 500 + 1.7 x 200 ) = 780 lbs .
Puw = 0.75 ( 1.4 x 64 psf x 13' ) = 874 lbs .
Pu = 1654 lbs .
( Pu + As f y ) ( 1.65 kips + 0.075 x 60 ksi )
Ase = = = 0.103 in 2
fy 60 ksi
( Pu + As f y ) 0.103 ( 60 )
a= = = 0.302quot;
0.85 f 'm b ( 0.85 x 2.0 ksi x 12quot; )
0.302quot;
c= = 0.355quot; < 1.2quot; neutral axis within face shell, treat as rectangular section
0.85

Mu=φMn = φAsefy(d - a/2)=0.8(0.103in2)(60ksi)(3.81in - 0.302in/2)=18.1 kip-in


Flexural Strength per UBC
Es 29,000
Em =750 f 'm = 1500ksi n= = = 19.3
Em 1500
Icr = nAse ( d − c )2 +
bc3
3
( )
= 19.3 0.103in2 ( 3.81− 0.355 )2 +
12( 0.355 )3
3
quot;
= 23.9 in4
2 2
5 Mcrh 5 ( Mu − Mcr )h
∆u = +
48 Em I g 48 Em Icr
12quot; x7.632
Mcr = fr Sg fr = 2.5( f 'm )0.5 = 112 psi Mcr = 0.112ksi ( ) = 13.1 k − in.
6

for simplicity, use gross section
even though partially grouted to avoid iteration, assume Mmax = Mu

5 ( 13.1 )( 20 x 12 )2 5 ( 18.1− 13.1 )( 20 x 12 )2
∆u = + = 0.118 + 0.837quot; = 0.955
quot; quot;
48 ( 1500)( 444 ) 48 ( 1500)( 23.9 )

w ( 20 )2 0.780 7.31 / 2 )
' ( quot; 0.955
Mu = u + +( 1.654)( )=181k − in. / 12
. quot;
8 12 12
wu
ws = = 17.8psf
wu=22.7 psf 0.75x1.7

Check Service Load Deflections per UBC

∆s < 0.007 h
5 M cr h 2 5 ( M s − M cr )h 2
∆s = +

w s h2
Ms = + Po e / 2 + ( Pw + Po ) ∆s
8
⎡ ( 17.8 psf )( 20 )2 ⎤ 700 ( 7.31quot; )
=⎢ ⎥ x 12 + + 1532 ∆s = 13 ,239 + 1532 ∆s ( lb . − in .)
⎢
⎣ 8 ⎥
⎦ 2

5 ( 13.239 + 1.532 ∆s − 13.1 )( 20 x 12 )2
∆s = 0.118quot; +
48 ( 1 ,500 )( 23.9 )
∆s = 0.19quot; < 0.007 h = 0.007 (20' x 12 ) = 1.68quot; ok )


Maximum Wind Load per MSJC
d = 3.82”
1.25”

w h2 P e
M s = s + os + ( Pws + Pos )∆s
8 2
#4 @ 32”
w s ( 20' )2
= x 12 + 700 lbs ( 3.66quot; ) + ( 1532 lbs ) ∆s
8
= 600 w s + 2562 lb − in . + 1532 ∆s

Determine Icr considering axial compression

k 2 + 2 n( ρ + α )k − 2 n( ρ + α ) = 0

0.075 in 2 P 1.532 k
n = 19.3 ρ= = 0.00164 α= = = 0.00104
12quot;×3.82quot; bdFs 12quot; × 3 .82quot; × 32 ksi

k 2 + 0.0104 k − 0.0104 = 0 k = 0.275 kd = 1.05quot; < face shell thickness, ok
k
j = 1 − = 0.908 jd = 3.47quot;
3
b( kd )3 12quot; ( 1.05quot; )3
I cr = + nAs ( d − kd )2 = + 19.3( 0.075 in 2 )( 3.82quot; −1.05quot; )2 = 15.7 in 4
3 3


Maximum Wind Load per MSJC
M s = As Fs + ( Pws + Pos ) jd = [( 0.075 × 32 ksi) + 1.532 ] 3.47quot; = 13.64 kip - in
0.600 w s + 2.56 kip - in . + 1.532 ∆s = 13.64 kip - in
w s = 18.47 − 2.55 ∆s ( w s is in psf)
2
5 M cr h 5 ( M s − M cr )h 2
∆s = +

5 ( 0.600 w s + 2.56 kip - in . + 1.532 ∆s − 13.1 )( 20 × 12 )2
∆s = 0.118quot; +
48 ( 1500 ksi)(15.7 in 4 )
∆s = 0.118quot; +0.153 w s + 0.652 + 0.390 ∆s − 3.34
∆s = 0.251 w s − 4.21

w s = 18.47 − 2.55 ∆s ( 0.251 w s − 4.21 ) w s = 17.8 psf

Note: same wind load as by UBC slender wall design procedure.
Should also check compressive stress with an axial force-moment interaction diagram


Strength Design of RM Shear Walls
UBC Requirements
UBC Sec. 2108.1.1: Strength procedure may be used as an alternative to Sec. 2107 for
design of reinforced hollow-unit masonry walls.
UBC Sec. 2108.1.2: Special inspection must be provided during construction. Prisms
should be tested or unit strength method should be used.
UBC Sec.2108.1.3: Shear wall design procedure
1. Required strength
A. earthquake loading: U = 1.4 (D+L+E) (12-1)
U = 0.9D + - 1.4E (12-2)
B. gravity loading: U = 1.4D + 1.7E (12-3)
C. wind loading: U = 0.75(1.4D + 1.7 L + 1.7W) (12-4)
U = 0.9D + - 1.3W (12-5)
D. earth pressure: U = 1.4D + 1.7L + 1.7H (12-6)
2. Design strength
φ = 0.65 for φPn > 0.1 f 'm Ae or φPn > 0.25 Pb (see next slide)
A. axial load and flexure
φ = 0.85 for φPn = 0
B. shear φ = 0 .60 for shear limit state
φ = 0.80 for flexure limit state


Definition of Balanced Axial Load, Pb
ε mu 0.85f’m
Cs1
a b = 0.85 c Cm = 0.85f’mbab
c
Cs2
Lw d
Pb
n.a.
Ts3

Ts4 = Asbalfy
ε s = ε y = 0.00207
b

assume ΣC si = ΣTsi so Pb = C m
emu
for solidly grouted walls : Pb = 0.85 f 'm bab where ab = 0.85 d
fy
( emu + )
Es


UBC Requirements

3. Design assumptions (same as for Slender Wall Design Procedure, UBC Sec. 2108.2.1.2)

1. equilibrium
2. strain compatibility
3. εmu = 0.003
4. fs = Esεs < fy
5. neglect masonry tensile strength
6. use rectangular stress block, k1 = 0.85, k3 = 0.85
7. 1500 psi < f’m < 4000 psi


UBC Requirements
4. Reinforcement per UBC Sec. 2108.2.5.2
Mn > Mcr
1. minimum reinforcement M ductile
ρ v + ρ h ≥ 0.002
ρ v and ρ h ≥ 0.0007 Mcr

spacing ≤ 4' −0quot; Mn < Mcr
nonductile
2. for flexural failure mode
Mn > = 1.8 Mcr for fully grouted wall ∆
Mn > = 3.0 Mcr for partially grouted wall

3. anchor all continuous reinforcement
4. As vertical > 1/2 As horizontal
5. maximum spacing of horizontal reinforcement within plastic hinge region = 3t or 24”

5. Axial strength (no flexure)
Po = 0.85 f’m(Ac-As) + fyAs Pu < = φ (0.80)Po


UBC Requirements

6. Shear Strength UBC Sec. 2108.2.5.5
1. maximum nominal shear:
M
Vn = 6.0 Ae f 'm ≤ 380 Ae for ≤ 0.25
Vd
M
= 4.0 Ae f 'm ≤ 250 Ae for ≥ 1.0 (Table 21 - J )
Vd
Amv t
2. for walls limited by shear strength:
Lw
Vn = Vm + Vs
where Vm = C d Amv f 'm (8 - 37) Vu
M
and C d = 2.4 for ≤ 0.25
Vd Amv = net area of masonry
M wall section bounded by
= 1.2 for ≥ 1.0 (Table 21 - K)
Vd wall thickness and length of
section in direction of shear


UBC Requirements
t
6. Shear Strength UBC Sec. 2108.2.5.5.2 (continued)

Vs = Amv ρ n f y (8 − 38)
Avertical plane
where ρ n = As horizontal / Avertical plane
h
As horizontal
Vs = Amv ( )fy
Avertical plane
As horizontal
As horizontal Lw
Vs = Lw t ( ) fy = ( As horizontal ) f y
ht h

Lw Lw Lw

As fy
h As fy
As fy


UBC Requirements
6. Shear Strength UBC Sec. 2108.2.5.5: continued

3. for walls limited by flexural strength:
within hinge region, distance of Lw above base:

V n = V s = Am ρ n f y (8 − 39)
(Vu determined at Lw/2 from base)

above hinge region:

V n = Vm + V s


UBC Requirements
Boundary Members: Sec.2108.2.5.6
1. Provide boundary members when the extreme fiber strain exceeds 0.0015.
2. The minimum length of boundary members shall be 3t.
3. Boundary members shall be confined with a minimum of #3 bars @ 8”
spacing, or equivalent confinement to develop an ultimate compressive masonry
strain equal to 0.006.

#3 @ 8”
wall
> 3t centroid
min.

t

0.0015
εmu > 0.0015

Section at Base of Wall


Example: Strength Design
Determine the maximum wind force, H, and design horizontal
reinforcement to develop the wall flexural strength.
Consider: zero vertical load and
5’-4”
H Pdead = 40 kips and Plive = 30 kips

8” concrete block, fully grouted
Grade 60 reinforcement , f’m= 1500 psi
from previous example:
10’-8”

Mn = 5 ,222 kip − in.
Mu = φ Mn = 0 .85( 5 ,222) = 4 ,439 kip − in.

check cracking moment per Eq. 8 - 30
M cr = f r S g f r = 4.0 f'm < 235 psi
f r = 4.0 1500 = 155 psi
64 2 M
M cr = ( 0.155 ksi)(7.63 × ) = 807 kip - in < n ok
7.63”

6 1.8
Mu H
4 - #8’s if flexure limit state exists : H u = = 34.7 kips H = u = 26.7 kips
128quot; 1.3


Shear Reinforcement (neglecting vertical force) U = 1.3W

shear design within Lw (5’-4”) of base:
L
Vn = Vs = Amv ρ n f y = ( w ) As horiz f y = ( 0.5 ) As horiz ( 60 ksi)
h
Vu = H u = 34.7 kips = φ Vn = 0.80[(0.5) (Ahoriz ) (60 ksi)]

As horiz = 1.45 in 2 smax = 24quot; use #4' s @ 8quot; for bottom 8 courses

As horiz provided = 8(0.20 in 2 ) = 1.60 in 2 > 1.45 in 2

shear design for top 5’-4” of wall:
Vn = Vm + Vs = C d Amv f'm + Am ρ n f y
L
= 1.2(7.63 x 64.00) 1500 + ( w )(As horiz )f y = 22.7 kips + (0.5)(As horiz )(60 ksi)
h
Vu = H u = 34.7 kips
= φ Vn = 0.80[22.7 + (0.5)(As horiz )(60 ksi)]

As horiz = 0.69 in 2 smax = 48quot; use #4' s @ 16quot; for top 8 courses

As horiz provided = 4(0.20 in ) = 0.80 in 2 > 0.69 in 2
2


Example:Shear Wall Strength Design
Confinement Reinforcement (neglecting vertical force)
Confinement requirements for vertical reinforcement per Sec. 2108.2.5.6

Mu = 4,439 kip-in.

5’-4”
7.63”

3t > 5.7” #8 #3 @ 8”
bottom 8 courses
11.5”

ε = 0.003
ε = 0.0015
5.75” Strain Diagram per Previous Example


Flexural Strength considering Vertical Loads
Case 1: Pu = 0.75(1.4 x 40 + 1.7 x 30) = 80.3 kips perhaps maximum flexural
capacity and critical for shear design

Case 2: Pu = 0.9(40) = 36.0 kips perhaps minimum flexural capacity and lowest Hu

capacity reduction factors

Pb = 0.85f'm bab
ε mu 0.003
ab = 0.85 d = 0.85 60quot; = 30.2quot;
fy 0.003 + 0.00207
ε mu +
Es
Pb = 0 .85( 1 .5 ksi)( 7 .63quot;)( 30 .2quot;) = 294 kips 0 .25 Pb = 73 .5 kips
considering reinforcement : Pb = −294 + 0 .79 in 2 ( − 60 .0 - 36 .0 + 20 .2 + 60 .0 ) = −306 kips

Case 1: Pu = 80.3 kips > 0.25 Pb = 73.5 kips φ = 0.65
36.0
Case 2: Pu = 36.0 kips < 0.25 Pb = 73.5 kips φ = 0.65 + ( )0.20 = 0.75
73.5


⎛ c − di ⎞
εi = ⎜ ⎟ ( − 0.003 ) f si = E s ε si < f y C m = 8.27 c
⎝ c ⎠

d1 = 4.0” d2 = 20.0” d3 = 44.0”
ε1 f1 Cs1 ε2 f2 Ts2 ε3 f3 Cs3 Cm Pn Mn
(ksi) (kips) (ksi) (kips) (ksi) (kips) (kips) (kips) (kip-in)

20.0 -0.00240 -60 -47.4 0 0 0 0.00360 60.0 47.4 -165 -118 6,983
15.0 -0.00220 -60 -47.4 0.00100 29.0 22.9 0.00580 60.0 47.4 -124 -54 6,126

16.8 -0.00229 -60 -47.4 0.00057 16.6 13.1 0.00185 53.8 42.5 -139 -83 6,463

13.1 -0.00208 -60 -47.4 0.00104 30.0 23.7 0.00708 60.0 47.4 -108 -37 5,794

0.85c
∑ M cl = C m (32.0quot; - ) + C sl (28.0quot; ) - Ts2 ( 12.0quot; ) + Ts3 ( 12.0quot; ) + Ts4 ( 28.0quot; )
2



Case 1: M u = φ M n = 0.65(6450) = 4,192 kip - in.
Axial Compressive Force, kips

140

6450 kip-in.
4,192
120 Hu = = 32.7 kips
(10.67 x 12)
100 Hu
H= = 25.7 kips governs
( 0.75 x 1.7)
5820 kip-in.

80 80.3 kips
Case 1
60 Case 2: M u = φ M n = 0.75(5820) = 4,365 kip - in.
40 4,365
Case 2 Hu = = 34.1 kips
36.0 kips (10.67 x 12)
20
Hu
H= = 26.2 kips
1.3
5500 6000 6500 7000
Moment, Mn kip-in. Hu = 34.1 kips (Case 2) ~ 34.7 kips (w/o vertical force).
Use same shear design as for first part of problem.

Mu = 4,365 kip-in. (Case 2) ~ 4,439 kip-in. (w/o vertical force).
Use same boundary members as for first part of problem.


Lecture 6 7 Rm Shear Walls

Empfohlen

Empfohlen

Weitere ähnliche Inhalte

Was ist angesagt?

Was ist angesagt? (20)

Andere mochten auch

Andere mochten auch (13)

Mehr von Teja Ande

Mehr von Teja Ande (20)

Kürzlich hochgeladen

Kürzlich hochgeladen (20)

Lecture 6 7 Rm Shear Walls