For UniKL Business School Student rare slide

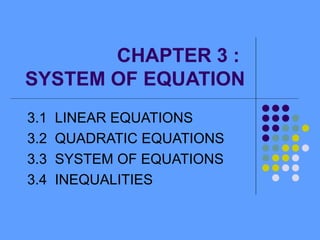
1. CHAPTER 3 :
SYSTEM OF EQUATION
3.1
3.2
3.3
3.4
LINEAR EQUATIONS
QUADRATIC EQUATIONS
SYSTEM OF EQUATIONS
INEQUALITIES

2. 3.1 LINEAR EQUATION
Introduction


An equation is simply a statement that 2
quantities are the same.
E.g. 45 = 9
+
LHS = RHS


LHS



RHS
A statement can be true or false is said
to be a conditional (or open) equation
Think of an equation as a
pair of perfectly balanced
as it is true for some values of the variable
old – fashioned scales
& not true for others.
Equation =
For example: 2x + 3 = 7 is true for x = 2
but is false for x = 8 or other values.
Any number that makes the equation true
is called a solution or root of the
equation.

3. 3.1 LINEAR EQUATION
Introduction



A statement such as 2 x + 22 = 2( x + 11) is called an
identity as it is true for all real numbers x.
While solving equation, there may be no solution,
one solution or there may be more than one
solution.
For example:
Equation
Solution
Comment
x + 9 = 12
x=3
Only one solution
x2 = 81
x = 9 or – 9
Two solutions
x=5+x
No solution
No solution
x2 – 16 = (x – 4)(x + 4)
All values of x
Equation is true for all
values of x.

4. 3.1 LINEAR EQUATION
Solving Linear equation with 1 variable



To solve an equation, we find the values of the
unknown that satisfy it.
How: Isolate the unknown / variable & solve its value.
A linear equation is an equation that can be written in
the form;
ax + b = 0

where a & b are real numbers.
Linear equations have only one root/ solution.

5. 3.1 LINEAR EQUATION
Solving Linear equation with 1 variable
x − 3 = 12
Add 3 to both sides
x − 3 + 3 = 12 + 3
x = 15
x
= 12
3
Multiply both sides by 3
x
3  = 3(12)
3
x = 36
x + 3 = 12
Subtract 3 from both sides
x + 3 − 3 = 12 − 3
x=9
3 x = 12
Perform the
same
operation
on both
sides
Divide both sides by 3
3 x 12
=
3
3
x=4
Always check
in the
original
equation

6. Example 1
Solve the equation x − 7 = 3 x − ( 6 x − 8 ).
Working
Step
x − 7 = 3x − 6x + 8
Parentheses removed
x − 7 = −3 x + 8
4x −7 = 8
4x =
15
15
x =
4
x – terms combined
3x added to both sides
7 added to both sides
Both sides divided by 4

7. PRACTICE 1
1. Solve the following equation : 2. Solve the given equation.
a) 5( x − 3 ) = 10
a) 6 + 4L = 5 − 3L
x 3−x
b) 3( 4 − n ) = −n
b) +
= 12
2
4
c) 1.5 x − 0.3( x − 4 ) = 6
2
x 36
c) x + 10 = +
d) 7 − 3(1 − 2 p ) = 4 + 2 p
3
5 5
3 − 5( 7 − 3V )
2x − 3 2
e) 2V =
d)
=
x +1 5
4

8. 3.2 QUADRATIC EQUATION
Introduction

A quadratic equation contains terms of the first and
second degree of unknown is given by;
2
ax + bx + c = 0


Standard
Standard
form
form
where a, b, c are real numbers and a ≠ 0.
A quadratic equation that contains terms of the 2nd
degree only of the unknown is called a pure
quadratic equation, can be expressed as ;
ax 2 + c = 0
A value of the unknown that will satisfy the equation
is called a solution or a root of the equation.

9. 3.2 QUADRATIC EQUATION
Solving quadratic equation
 All quadratic equations have two roots .
 In pure quadratic equation , the roots are equal but of
opposite sign. It can solve by extraction of the root.
 There are two methods of solving complete quadratic
equation;
 By factorization
 By using quadratic formula

10. Example 2
Solve the equation :
a) x 2 = 9
x = 9 = ±3
b) x 2 − 16 = 0
x 2 = 16
x = 16 = ±4
c) 5R 2 − 89 = 0
5R 2 = 89
89
R2 =
5
R=
89
= ±4.219
5
d) x 2 + 36 = 0
x 2 = −36
x = − 36
x = − 1 36
x = ±i 6
e) ( 2 x − 5 ) + 5 = 3
2
Where
Where
−1 = i

11. 3.2 QUADRATIC EQUATION
Solving quadratic equation Factorization
 The expression ax 2 + bx + c is written as the
product of two factors.
 If the product of 2 factors is zero, then one of those
factors must be zero. Thus if mn = 0 then either
m = 0 or n = 0.
 Only be used if the quadratic expression can be
factorized completely.

12. Example 3
Solve the quadratic equation :
a) x 2 − 6 x = 0
x ( x − 6) = 0
x = 0 or x − 6 = 0
x=6
b) 5 x 2 − 2 x = 0
x ( 5 x − 2) = 0
x = 0 or 5 x − 2 = 0
x=
2
5
c) x 2 + x − 12 = 0
(x+4)
x
4
= 4x
x
-3
= -3x
-12
=x
( x + 4)( x − 3 ) = 0
x + 4 = 0 or x − 3 = 0
x = −4
x =3
(x-3)
x2

13. Example 3
Solve the quadratic equation :
2
d) x + 7 x + 12 = 0
( x + 4)( x + 3) = 0
x + 4 = 0 or x + 3 = 0
x = −4
x = −3
e) x 2 + 6 x − 7 = 0
( x − 1)( x + 7) = 0
x − 1 = 0 or x + 7 = 0
x =1
x = −7
f) x ( x − 1) = 6
x2 − x − 6 = 0
( x + 2)( x − 3) = 0
x + 2 = 0 or x − 3 = 0
x = −2
x =3
(x+4)
x
4
= 4x
x
3
= 3x
12
= 7x
(x+3)
x2

14. PRACTICE 2
Solve the quadratic equation :
a) x 2 + 5 x − 14 = 0
b) 3 x 2 + 10 x = 8
c) 3 x 2 − 5 x − 2 = 0
d) 6 x 2 − 11x − 35 = 0
e) ( x − 2)( x + 4 ) = 16

15. 3.2 QUADRATIC EQUATION
Solving quadratic equation –
Quadratic Formula
 For any quadratic equation ax2 + bx + c = 0, the
solutions for x can be found by using the quadratic
formula:
− b ± b 2 − 4ac
x=
2a
 The quantity b2 – 4ac is called discriminant of the
quadratic equation that characterize the solution of the
quadratic equations:
 If b2 – 4ac = 0 one real solution
 If b2 – 4ac > 0  2 real and unequal solutions
 If b2 – 4ac < 0  no real but 2 imaginary solutions
(complex number)

16. Example 4
1. Solve the equation 2k 2 + 8k − 5 = 0 by using the quadratic formula
Step 1
Identify a, b & c, by comparing with ax2 + bx + c = 0.
a = 2, b = 8, c = -5
Step 2
− b ± b 2 − 4ac
Substitute into the formula: k =
2a
− 8 ± 8 2 − 4( 2)( − 5 )
=
2( 2)
=
Step 3
The solutions are:
− 8 + 104
4
= 0.5495
k1 =
− 8 ± 104
4
− 8 − 104
4
= −4.5495
k2 =
b22--4ac > 0,
b 4ac > 0,
2 real &
2 real &
unequal
unequal
roots
roots

17. Example 4
2. Solve the equation 4 p 2 − 12 p + 9 = 0 by using the quadratic formula
Step 1
Identify a, b & c, by comparing with ax2 + bx + c = 0.
a = 4, b = -12, c = 9
Step 2
− b ± b 2 − 4ac
Substitute into the formula: p =
2a
=
=
Step 3
The solution is:
12 3
p=
=
8 2
− ( − 12) ±
12 ± 0
8
( − 12) 2 − 4( 4)( 9 )
2( 4 )
b22--4ac = 0,
b 4ac = 0,
1 real root
1 real root

18. Example 4
3. Solve the equation 3k 2 − 5k + 4 = 0 by using the quadratic formula
Step 1
Identify a, b & c, by comparing with ax2 + bx + c = 0.
a = 3, b = -5, c = 4
Step 2
− b ± b 2 − 4ac
Substitute into the formula: k =
2a
b22--4ac < 0,
b 4ac < 0,
no real, 2
no real, 2
imaginary roots
imaginary roots
Step 3
=
5 + i 23
6
= 0.83 + i 0.8
( − 5 ) 2 − 4( 3)( 4)
2( 3 )
=
5 ± − 23
6
=
The solutions are:
k1 =
− ( − 5) ±
5 ± − 1 23 5 ± i 23
=
6
6
5 − i 23
6
= 0.83 − i 0.8
k2 =

19. PRACTICE 3
Solve the quadratic equation below by
using quadratic formula :
a) x 2 + 7 x − 8 = 0
b) 9 x 2 + 49 = 42 x
c) 3 x 2 + 7 x + 3 = 0
3
d)
= 2R
5−R
e) s 2 = 9 + s (1 − 2s )

20. 3.3 SYSTEMS OF EQUATION
Introduction
 A system of equations contains 2 or more equations.
Each equation contains 1 or more variables.
a1x + b1y = c1
a2 x + b2 y = c 2
Where a & b are
Where a & b are
coefficients of xx& y,
coefficients of & y,
ccis a constant.
is a constant.
 A solution of the system is any pair of values (x , y)
that satisfies the both equations.
 2 methods that are usually used:
Substitution method
Elimination method

21. 3.3 SYSTEMS OF EQUATION
Substitution Method
Step for solving by substitution:
 Pick 1 of the equation & solve for 1 of the
variables in terms of the remaining variables.
 Substitute the result in the remaining
equations.
 If 1 equation in 1 variable results, solve the
equation. Otherwise, repeat Step 1 – 3 again.
 Find the values of the remaining variables by
back – substitution.
 Check the solution found.

22. Example 5 a
Solve the following system of equations
x − 3 y = 6 → Eq 1
Solution:
2 x + 3 y = 3 → Eq 2
Step 1 From Eq 1, make x as subject: x = 6 + 3 y
Step 2 Substitute x in Eq 2 : 2( 6 + 3 y ) + 3 y = 3
Step 3 Solve for y :
12 + 6 y + 3 y = 3
9 y = −9
y = −1
Step 4 Substitute y in Eq 1, to solve for x: x = 6 + 3( −1) = 3
Step 5 Check the solution : Eq 1, 3 – 3(-1) = 6
Eq 2, 2(3) + 3(-1) = 3

23. Example 5 b
Solve the following system of equations
x + 2y = 8 → Eq 1
The solution is
The solution is
Solution:
the intersection
the intersection
3 y − 4 x = 1 → Eq 2
Step 1 From Eq 1, make x as subject: x = 8 − 2y
Step 2 Substitute x in Eq 2 : 3 y − 4( 8 − 2 y ) = 1
Step 3 Solve for y :
3 y − 32 + 8 y = 1
11y = 33
y =3
Step 4 Substitute y in Eq 1, to solve for x:
x = 8 − 2( 3 ) = 2
Step 5 Check the solution :
Eq 1; 2 + 2(3) = 8
Eq 2; 3(3) – 4(2) = 1
point (2,3)
point (2,3)

24. Example 5 c
Solve the following system of equations
The lines are
4 x + 2y = 8 → Eq 1
The lines are
Solution:
parallel & no
parallel & no
2 x + y = 5 → Eq 2
Step 1 From Eq 2, make y as subject: y = 5 − 2 x
Step 2 Substitute y in Eq 1 : 4 x + 2( 5 − 2 x ) = 8
4 x + 10 − 4 x = 8
10 = 8
Step 3 The result is a false statement.
Therefore the system is inconsistent.
It means that there is no solutions for
this system of equations
intersection
intersection

25. Example 5 d
Solve the following system of equations
Solution:
2 x + y = 4 → Eq 1
−6 x − 3 y = −12 → Eq 2
The lines is
The lines is
coincide, the
coincide, the
systems has
systems has
infinitely many
infinitely many
solution
solution
Step 1 From Eq 1, make y as subject: y = 4 − 2 x
Step 2 Substitute y in Eq 2 :
− 6 x − 3( 4 − 2 x ) = − 12
− 6 x − 12 + 6 x = − 12
− 12 = − 12
Step 3 The result is a true statement.
Therefore the system is
dependent. It means that there is
no unique solutions can be
determined.

26. 3.3 SYSTEMS OF EQUATION
Elimination Method
Step for solving by elimination:





Write x’s, y’s & numbers in the same order in both
equations.
Compare x’s & y’s in the 2 equations & decide which
unknown is easier to eliminate. Make sure the number
of this unknown is the same in both equations.
Eliminate the equal terms by adding or subtracting the 2
equations
Solve the resulting equation & substitute in the simpler
original equation in order to find the value of the other
unknown.
Check the solution found.

27. Example 6 a
Solution:
Solve the following system of equations
7 y = 6 − 4 x → Eq1
3 x = 2y + 19 → Eq 2
Multiply by a
Multiply by a
constants to
constants to
get same
get same
coefficient of x
coefficient of x
Step 1 Rearrange the eq: 4 x + 7 y = 6 →Eq1
3 x − 2 y = 19 →Eq 2
Step 2 To eliminate x, Eq1 multiply by 3, Eq 2 multiply by 4
12 x + 21y = 18 → Eq 3
12 x − 8 y = 76 → Eq 4
Step 3 Eq 3 – Eq 4, solve for y : 21y − ( − 8 y ) = 18 − 76
29 y = −58
y = −2
Step 4 Substitute y in Eq 1, to solve for x: 4 x = 6 − 7( − 2)
20
x=
=5
4
Step 5 Check the solution :

28. Example 6 b
Solve the following system of equations
3 x − 2y = 4 → Eq1
x + 3 y = 2 → Eq 2
Step 1 To eliminate x, Eq2 multiply by 3;
3 x − 2y = 4 → Eq1
3 x + 9 y = 6 → Eq 3
Solution:
Step 2 Eq 1 – Eq 3, solve for y : − 2 y − 9 y = 4 − 6
−11y = −2
2
y =
11
2
Step 3 Substitute y in Eq 2, to solve for x: x + 3  = 2
 11 
11x + 6 = 22
Step 4 Check the solution :
x=
16
11

29. PRACTICE 4
Solve the following system by using
A. Substitution method
B. Elimination method
1) 2 x − y = 5
1) − 2 x + 2y = 5
x + 6y = 1
6 x + 2y = −5
2) 9m − 6n = 12
2) 2m + 6n = −3
2m + 6n = 4
− 6m − 18n = 5
3)
2R + S = 4
3) − 5K + 2L = −4
− 6R − 3S = −12
10K + 6L = 3
4) x 2 + y 2 = 4
x+y =2
4) y = x 2 + 2 x + 2
y = 3x + 8

30. 3.4 INEQUALITY
Introduction
 An inequality is a statement involving 2 expressions
separated by any of the inequality symbols below.
Inequality
Meaning
a>b
a is greater than b
a<b
a is less than b
a≥b
a is greater than or equal to b
a≤b
a is less than or equal to b
 A real number is a solution of an inequality involving
a variable if a true statement is obtained when the
variable is replaced by the number.
 The set of all real numbers satisfy the inequality is
called the solution set.

31. 3.4 INEQUALITY
Inequality, Interval Notation & Line Graph
Inequality
Notation
Interval
Notation
a≤x≤b
a<x≤b
a≤x<b
a<x<b
x≥b
x>b
x≤a
x<a
[ a, b ]
( a, b ]
[ a, b )
( a, b )
[ b, ∞ )
( b, ∞ )
( -∞, a ]
( -∞, a )
a
Graph
b

32. 3.4 INEQUALITY
Properties of Inequality
Properties
Example
Let a, b & c be any real number
If a < b and b < c, then a < c If 2 < 3 & 3 < 8 so 2 < 8
If a < b, then a ± c < b ± c
5 < 7, 5 + 3 < 7 + 3, 8 < 10
5 < 7, 5 - 3 < 7 - 3, 2 < 4
If a < b, c > 0 then ac < bc
5 < 7, 5(2) < 7(2), 10 < 14
If a < b, c < 0 then ac > bc
5 < 7, 5(-2) > 7(-2), -10 > -14
If a < b, c > 0 then a/c < b/c
5 < 7, 5/2 < 7/2, 2.5 < 3.5
If a < b, c < 0 then a/c > b/c
5 < 7, 5/(-2) > 7/(-2), -2.5 > -3.5
 Similar properties hold if < between a and b is replaced
by ≥ , > , or ≤ .

33. Example 7
Solve each of the following inequalities :
a) 3 x + 10 < 4 x − 7
b) 4 x + 5 ≥ 2 x + 9
3 x − 4 x < −7 − 10
− x < −17
Divide by
Divide by
–ve no , , ∴ x > 17
–ve no
reverse
reverse
sign
sign
c) 3 − 7 x < 2 x + 21
d)
6 x + 4 2x − 1
>
5
3

34. Example 8
Solve each of the following inequalities :
a) − 11 < 3 x − 2 < 4
− 11 < 3 x − 2
and
3x − 2 < 4
− 9 < 3x
3x < 6
−3< x
x<2
Therefore − 3 < x < 2 or x = { − 2, − 1, 0,1}
b) 10 − 3 x ≤ 2 x − 7 < x − 13
10 − 3 x ≤ 2 x − 7
and
− 5 x ≤ −17
Solution
Solution
set
set
2 x − 7 < x − 13
x < −6
17
x≥
5
Therefore there is no solution.
Impossible to have x that
Impossible to have x that
satisfies both inequalities
satisfies both inequalities

35. PRACTICE 5
1. If x is an integer, find the solution set for 3 ≤ x + 1 ≤ 5.
1
2. Determine the range of values of x satisfying < −4.
x
3. Solve the inequality :
a) 3( 2 x + 1) − 5( x − 1) ≥ 7 x
b) − 1 ≤ 2 x − 5 < 7
4. Find the minimum cost C (in dollars) given that
5(C − 25 ) ≥ 1.75 + 2.5C
5. Find the maximum profit P (in dollars) given that
6( P − 2500 ) ≤ 4( P + 2400 )