Solving Simultaneous Equations Using Graphs and Algebra
•Als PPT, PDF herunterladen•
25 gefällt mir•27,672 views
Simultaneous equations are two or more equations with the same unknown variables. There are two main methods to solve simultaneous equations: 1) Using graphs - make a table of values, plot the equations on a graph, and find where the graphs intersect. 2) Using algebra - organize the equations, make coefficients equal, eliminate a variable, solve the resulting equation, substitute values back into the original equations, and check the answer. The algebraic method follows the steps of NO MESS: Organize, Make equal, Eliminate, Solve, Substitute.
Melden
Teilen
Melden
Teilen
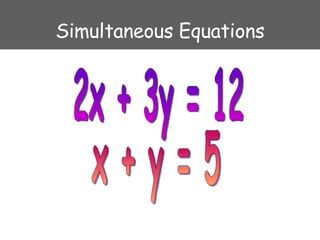
Weitere ähnliche Inhalte
Was ist angesagt?
Was ist angesagt? (20)
solving quadratic equations using quadratic formula

solving quadratic equations using quadratic formula
Andere mochten auch
Andere mochten auch (20)
Secondary 2 Mathematics-Simultaneous Linear Equation in 2 Unknowns Worked Exa...

Secondary 2 Mathematics-Simultaneous Linear Equation in 2 Unknowns Worked Exa...
Ähnlich wie Solving Simultaneous Equations Using Graphs and Algebra
Contextualized Lesson Plan in Math 7 Linear Equation in One Variable

Contextualized Lesson Plan in Math 7 Linear Equation in One VariableDepartment of Education - Philippines
Ähnlich wie Solving Simultaneous Equations Using Graphs and Algebra (20)
Contextualized Lesson Plan in Math 7 Linear Equation in One Variable

Contextualized Lesson Plan in Math 7 Linear Equation in One Variable
Tricks to remember the quadratic equation.ACTION RESEARCH ON MATHS

Tricks to remember the quadratic equation.ACTION RESEARCH ON MATHS
Chapter 3. linear equation and linear equalities in one variables

Chapter 3. linear equation and linear equalities in one variables
Mehr von Lois Lindemann
Mehr von Lois Lindemann (9)
Kürzlich hochgeladen
Kürzlich hochgeladen (20)
FULL ENJOY 🔝 8264348440 🔝 Call Girls in Diplomatic Enclave | Delhi

FULL ENJOY 🔝 8264348440 🔝 Call Girls in Diplomatic Enclave | Delhi
[2024]Digital Global Overview Report 2024 Meltwater.pdf![[2024]Digital Global Overview Report 2024 Meltwater.pdf](data:image/gif;base64,R0lGODlhAQABAIAAAAAAAP///yH5BAEAAAAALAAAAAABAAEAAAIBRAA7)
![[2024]Digital Global Overview Report 2024 Meltwater.pdf](data:image/gif;base64,R0lGODlhAQABAIAAAAAAAP///yH5BAEAAAAALAAAAAABAAEAAAIBRAA7)
[2024]Digital Global Overview Report 2024 Meltwater.pdf
SQL Database Design For Developers at php[tek] 2024![SQL Database Design For Developers at php[tek] 2024](data:image/gif;base64,R0lGODlhAQABAIAAAAAAAP///yH5BAEAAAAALAAAAAABAAEAAAIBRAA7)
![SQL Database Design For Developers at php[tek] 2024](data:image/gif;base64,R0lGODlhAQABAIAAAAAAAP///yH5BAEAAAAALAAAAAABAAEAAAIBRAA7)
SQL Database Design For Developers at php[tek] 2024
Swan(sea) Song – personal research during my six years at Swansea ... and bey...

Swan(sea) Song – personal research during my six years at Swansea ... and bey...
08448380779 Call Girls In Civil Lines Women Seeking Men

08448380779 Call Girls In Civil Lines Women Seeking Men
Transforming Data Streams with Kafka Connect: An Introduction to Single Messa...

Transforming Data Streams with Kafka Connect: An Introduction to Single Messa...
How to Troubleshoot Apps for the Modern Connected Worker

How to Troubleshoot Apps for the Modern Connected Worker
Tech-Forward - Achieving Business Readiness For Copilot in Microsoft 365

Tech-Forward - Achieving Business Readiness For Copilot in Microsoft 365
Injustice - Developers Among Us (SciFiDevCon 2024)

Injustice - Developers Among Us (SciFiDevCon 2024)
08448380779 Call Girls In Friends Colony Women Seeking Men

08448380779 Call Girls In Friends Colony Women Seeking Men
Mastering MySQL Database Architecture: Deep Dive into MySQL Shell and MySQL R...

Mastering MySQL Database Architecture: Deep Dive into MySQL Shell and MySQL R...
Presentation on how to chat with PDF using ChatGPT code interpreter

Presentation on how to chat with PDF using ChatGPT code interpreter
From Event to Action: Accelerate Your Decision Making with Real-Time Automation

From Event to Action: Accelerate Your Decision Making with Real-Time Automation
08448380779 Call Girls In Greater Kailash - I Women Seeking Men

08448380779 Call Girls In Greater Kailash - I Women Seeking Men
Unblocking The Main Thread Solving ANRs and Frozen Frames

Unblocking The Main Thread Solving ANRs and Frozen Frames
Solving Simultaneous Equations Using Graphs and Algebra
- 1. Simultaneous Equations 2x + 3y = 12 x + y = 5
- 11. 2. Draw the two graphs:
- 12. 3. Write down the x and y values where the graphs cross : The lines cross where x = 2 and y =1
